Implicite Crowding Avoidance
One of the interesting properties of the FPD relation is the implicit handling of crowding. The following movie will demonstrate this. We can see 10 points, randomly moving around in the objective space spanned by the two functions
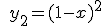
with x from [0,1]. The size of each point's disc is related to the ranking value of this point within the set of all ten points. The smaller the disc, the more this point is not dominated by the other points in the set. In terms of the multi-objective GA that we will study later: the smaller the disc, the more like is this point selected for mating. It can be seen in the movie that the points become smaller, as they move apart from other points. Once two points are approaching each other, the corresponding discs increase to the maximum size.
Movie: FPD Ranking Values
< FPD.Definition | FPD.FPD | FPD.Motivation >